Methods for solving the simplest logarithmic equations. Log formulas

As you know, when multiplying expressions with powers, their exponents always add up (a b * a c = a b + c). This mathematical law was derived by Archimedes, and later, in the 8th century, the mathematician Virasen created a table of integer indicators. It was they who served for the further discovery of logarithms. Examples of using this function can be found almost everywhere where it is required to simplify cumbersome multiplication to simple addition. If you spend 10 minutes reading this article, we will explain to you what logarithms are and how to work with them. Simple and accessible language.

Definition in mathematics

The logarithm is an expression of the following form: log a b=c, that is, the logarithm of any non-negative number (that is, any positive) "b" by its base "a" is considered the power of "c", to which the base "a" must be raised, so that in the end get the value "b". Let's analyze the logarithm using examples, let's say there is an expression log 2 8. How to find the answer? It's very simple, you need to find such a degree that from 2 to the required degree you get 8. Having done some calculations in your mind, we get the number 3! And rightly so, because 2 to the power of 3 gives the number 8 in the answer.

Varieties of logarithms

For many pupils and students, this topic seems complicated and incomprehensible, but in fact, logarithms are not so scary, the main thing is to understand their general meaning and remember their properties and some rules. There are three distinct kinds of logarithmic expressions:

Natural logarithm ln a, where the base is the Euler number (e = 2.7).
Decimal a, where the base is 10.
The logarithm of any number b to the base a>1.

Each of them is solved in a standard way, including simplification, reduction and subsequent reduction to one logarithm using logarithmic theorems. To obtain the correct values of logarithms, one should remember their properties and the order of actions in their decisions.

Rules and some restrictions

In mathematics, there are several rules-limitations that are accepted as an axiom, that is, they are not subject to discussion and are true. For example, it is impossible to divide numbers by zero, and it is also impossible to extract the root of an even degree from negative numbers. Logarithms also have their own rules, following which you can easily learn how to work even with long and capacious logarithmic expressions:

the base "a" must always be greater than zero, and at the same time not be equal to 1, otherwise the expression will lose its meaning, because "1" and "0" to any degree are always equal to their values;
if a > 0, then a b > 0, it turns out that "c" must be greater than zero.

How to solve logarithms?

For example, given the task to find the answer to the equation 10 x \u003d 100. It is very easy, you need to choose such a power by raising the number ten to which we get 100. This, of course, is 10 2 \u003d 100.

Now let's represent this expression as a logarithmic one. We get log 10 100 = 2. When solving logarithms, all actions practically converge to finding the degree to which the base of the logarithm must be entered in order to obtain a given number.

To accurately determine the value of an unknown degree, you must learn how to work with a table of degrees. It looks like this:

As you can see, some exponents can be guessed intuitively if you have a technical mindset and knowledge of the multiplication table. However, for large values you need a table of degrees. It can be used even by those who do not understand anything at all in complex mathematical topics. The left column contains numbers (base a), the top row of numbers is the value of the power c, to which the number a is raised. At the intersection in the cells, the values of the numbers are determined, which are the answer (a c =b). Let's take, for example, the very first cell with the number 10 and square it, we get the value 100, which is indicated at the intersection of our two cells. Everything is so simple and easy that even the most real humanist will understand!

Equations and inequalities

It turns out that under certain conditions, the exponent is the logarithm. Therefore, any mathematical numerical expressions can be written as a logarithmic equation. For example, 3 4 =81 can be written as the logarithm of 81 to base 3, which is four (log 3 81 = 4). For negative powers, the rules are the same: 2 -5 = 1/32 we write as a logarithm, we get log 2 (1/32) = -5. One of the most fascinating sections of mathematics is the topic of "logarithms". We will consider examples and solutions of equations a little lower, immediately after studying their properties. Now let's look at what inequalities look like and how to distinguish them from equations.

An expression of the following form is given: log 2 (x-1) > 3 - it is logarithmic inequality, since the unknown value "x" is under the sign of the logarithm. And also in the expression two quantities are compared: the logarithm of the desired number in base two is greater than the number three.

The most important difference between logarithmic equations and inequalities is that equations with logarithms (for example, the logarithm of 2 x = √9) imply one or more specific numerical values in the answer, while when solving the inequality, both the range of acceptable values and the points breaking this function. As a consequence, the answer is not a simple set of individual numbers, as in the answer of the equation, but a continuous series or set of numbers.

Basic theorems about logarithms

When solving primitive tasks on finding the values of the logarithm, its properties may not be known. However, when it comes to logarithmic equations or inequalities, first of all, it is necessary to clearly understand and apply in practice all the basic properties of logarithms. We will get acquainted with examples of equations later, let's first analyze each property in more detail.

The basic identity looks like this: a logaB =B. It only applies if a is greater than 0, not equal to one, and B is greater than zero.
The logarithm of the product can be represented in the following formula: log d (s 1 * s 2) = log d s 1 + log d s 2. In this case, the prerequisite is: d, s 1 and s 2 > 0; a≠1. You can give a proof for this formula of logarithms, with examples and a solution. Let log a s 1 = f 1 and log a s 2 = f 2 , then a f1 = s 1 , a f2 = s 2. We get that s 1 *s 2 = a f1 *a f2 = a f1+f2 (degree properties ), and further by definition: log a (s 1 *s 2)= f 1 + f 2 = log a s1 + log a s 2, which was to be proved.
The logarithm of the quotient looks like this: log a (s 1 / s 2) = log a s 1 - log a s 2.
The theorem in the form of a formula takes the following form: log a q b n = n/q log a b.

This formula is called "property of the degree of the logarithm". It resembles the properties of ordinary degrees, and it is not surprising, because all mathematics rests on regular postulates. Let's look at the proof.

Let log a b \u003d t, it turns out a t \u003d b. If you raise both parts to the power m: a tn = b n ;

but since a tn = (a q) nt/q = b n , hence log a q b n = (n*t)/t, then log a q b n = n/q log a b. The theorem has been proven.

Examples of problems and inequalities

The most common types of logarithm problems are examples of equations and inequalities. They are found in almost all problem books, and are also included in the mandatory part of exams in mathematics. For admission to the university or passing entrance examinations in mathematics, you need to know how to solve such problems correctly.

Unfortunately, there is no single plan or scheme for solving and determining the unknown value of the logarithm, however, certain rules can be applied to each mathematical inequality or logarithmic equation. First of all, you should find out whether the expression can be simplified or reduced to general view. You can simplify long logarithmic expressions if you use their properties correctly. Let's get to know them soon.

When deciding logarithmic equations, it is necessary to determine what kind of logarithm we have before us: an example of an expression may contain a natural logarithm or a decimal one.

Here are examples ln100, ln1026. Their solution boils down to the fact that you need to determine the degree to which the base 10 will be equal to 100 and 1026, respectively. For solutions natural logarithms one must apply logarithmic identities or their properties. Let's look at examples of solving logarithmic problems of various types.

How to Use Logarithm Formulas: With Examples and Solutions

So, let's look at examples of using the main theorems on logarithms.

The property of the logarithm of the product can be used in tasks where it is necessary to expand great importance numbers b into simpler factors. For example, log 2 4 + log 2 128 = log 2 (4*128) = log 2 512. The answer is 9.
log 4 8 = log 2 2 2 3 = 3/2 log 2 2 = 1.5 - as you can see, using the fourth property of the degree of the logarithm, we managed to solve at first glance a complex and unsolvable expression. It is only necessary to factorize the base and then take the exponent values out of the sign of the logarithm.

Tasks from the exam

Logarithms are often found in entrance exams, especially a lot of logarithmic problems in the Unified State Exam (state exam for all school graduates). Usually these tasks are present not only in part A (the easiest test part of the exam), but also in part C (the most difficult and voluminous tasks). The exam implies an accurate and perfect knowledge of the topic "Natural logarithms".

Examples and problem solving are taken from the official versions of the exam. Let's see how such tasks are solved.

Given log 2 (2x-1) = 4. Solution:
let's rewrite the expression, simplifying it a little log 2 (2x-1) = 2 2 , by the definition of the logarithm we get that 2x-1 = 2 4 , therefore 2x = 17; x = 8.5.

All logarithms are best reduced to the same base so that the solution is not cumbersome and confusing.
All expressions under the sign of the logarithm are indicated as positive, therefore, when taking out the exponent of the exponent of the expression, which is under the sign of the logarithm and as its base, the expression remaining under the logarithm must be positive.

Logarithmic expressions, solution of examples. In this article, we will consider problems related to solving logarithms. The tasks raise the question of finding the value of the expression. It should be noted that the concept of the logarithm is used in many tasks and it is extremely important to understand its meaning. As for the USE, the logarithm is used in solving equations, in applied problems, and also in tasks related to the study of functions.

Here are examples to understand the very meaning of the logarithm:

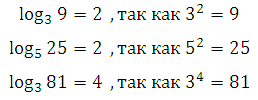
Basic logarithmic identity:

Properties of logarithms that you must always remember:

*The logarithm of the product is equal to the sum of the logarithms of the factors.

* * *

* The logarithm of the quotient (fraction) is equal to the difference of the logarithms of the factors.

* * *

* The logarithm of the degree is equal to the product of the exponent and the logarithm of its base.

* * *

*Transition to new base

* * *

More properties:

* * *

Computing logarithms is closely related to using the properties of exponents.

We list some of them:

The essence of this property is that when transferring the numerator to the denominator and vice versa, the sign of the exponent changes to the opposite. For example:

Consequence of this property:

* * *

When raising a power to a power, the base remains the same, but the exponents are multiplied.

* * *

As you can see, the very concept of the logarithm is simple. The main thing is that good practice is needed, which gives a certain skill. Certainly knowledge of formulas is obligatory. If the skill in the transformation of elementary logarithms is not formed, then when solving simple tasks it is easy to make a mistake.

Practice, solve the simplest examples from the math course first, then move on to more complex ones. In the future, I will definitely show how the “ugly” logarithms are solved, there will be no such ones at the exam, but they are of interest, do not miss it!

That's all! Good luck to you!

Sincerely, Alexander Krutitskikh

P.S: I would be grateful if you tell about the site in social networks.

Logarithmic equation called an equation in which the unknown (x) and expressions with it are under the sign logarithmic function. Solving logarithmic equations assumes that you are already familiar with and .
How to solve logarithmic equations?

The simplest equation is log a x = b, where a and b are some numbers, x is an unknown.
Solving the logarithmic equation is x = a b provided: a > 0, a 1.

It should be noted that if x is somewhere outside the logarithm, for example log 2 x \u003d x-2, then such an equation is already called mixed and a special approach is needed to solve it.

The ideal case is when you come across an equation in which only numbers are under the sign of the logarithm, for example x + 2 \u003d log 2 2. Here it is enough to know the properties of logarithms to solve it. But that kind of luck doesn't happen often, so get ready for more difficult stuff.

But first, after all, let's start with simple equations. To solve them, it is desirable to have the most general idea of the logarithm.

Solving simple logarithmic equations

These include equations like log 2 x \u003d log 2 16. It can be seen with the naked eye that by omitting the sign of the logarithm we get x \u003d 16.

In order to solve a more complex logarithmic equation, it is usually led to the solution of an ordinary algebraic equation or to the solution of the simplest logarithmic equation log a x = b. In the simplest equations, this occurs in one movement, which is why they are called the simplest.

The above method of dropping logarithms is one of the main ways to solve logarithmic equations and inequalities. In mathematics, this operation is called potentiation. There are certain rules or restrictions for this kind of operations:

logarithms have the same numerical bases
logarithms in both parts of the equation are free, i.e. without any coefficients and other various kinds of expressions.

Let's say in the equation log 2 x \u003d 2log 2 (1- x), potentiation is not applicable - the coefficient 2 on the right does not allow. In the following example, log 2 x + log 2 (1 - x) = log 2 (1 + x) one of the restrictions is also not satisfied - there are two logarithms on the left. That would be one - a completely different matter!

In general, you can remove logarithms only if the equation has the form:

log a(...) = log a(...)

Absolutely any expressions can be in brackets, this absolutely does not affect the potentiation operation. And after the elimination of logarithms, a simpler equation will remain - linear, quadratic, exponential, etc., which you already, I hope, know how to solve.

Let's take another example:

log 3 (2x-5) = log 3 x

Applying potentiation, we get:

log 3 (2x-1) = 2

Based on the definition of the logarithm, namely, that the logarithm is the number to which the base must be raised in order to obtain an expression that is under the sign of the logarithm, i.e. (4x-1), we get:

Again, we got a nice answer. Here we did without the elimination of logarithms, but potentiation is applicable here too, because the logarithm can be made from any number, and exactly the one that we need. This method is very helpful in solving logarithmic equations and especially inequalities.

Let's solve our logarithmic equation log 3 (2x-1) = 2 using potentiation:

Let's represent the number 2 as a logarithm, for example, such log 3 9, because 3 2 =9.

Then log 3 (2x-1) = log 3 9 and again we get the same equation 2x-1 = 9. I hope everything is clear.

So we looked at how to solve the simplest logarithmic equations, which are actually very important, because solution of logarithmic equations, even the most terrible and twisted ones, in the end always comes down to solving the simplest equations.

In everything we have done above, we have overlooked one very important point which will play a decisive role in the future. The fact is that the solution of any logarithmic equation, even the most elementary one, consists of two equivalent parts. The first is the solution of the equation itself, the second is work with the area of admissible values (ODV). That's just the first part we have mastered. In the above examples, the ODD does not affect the answer in any way, so we did not consider it.

Let's take another example:

log 3 (x 2 -3) = log 3 (2x)

Outwardly, this equation is no different from the elementary one, which is very successfully solved. But it is not so. No, of course we will solve it, but most likely it will be wrong, because there is a small ambush in it, into which both C students and honors students immediately fall into. Let's take a closer look at it.

Suppose you need to find the root of the equation or the sum of the roots, if there are several:

log 3 (x 2 -3) = log 3 (2x)

We apply potentiation, here it is permissible. As a result, we get the usual quadratic equation.

We find the roots of the equation:

There are two roots.

Answer: 3 and -1

At first glance, everything is correct. But let's check the result and substitute it into the original equation.

Let's start with x 1 = 3:

log 3 6 = log 3 6

The check was successful, now the queue x 2 = -1:

log 3 (-2) = log 3 (-2)

Yes, stop! Externally, everything is perfect. One moment - there are no logarithms from negative numbers! And this means that the root x \u003d -1 is not suitable for solving our equation. And therefore the correct answer will be 3, not 2, as we wrote.

It was here that the ODZ played its fatal role, which we forgot about.

Let me remind you that under the area of admissible values, such values \u200b\u200bof x are accepted that are allowed or make sense for the original example.

Without ODZ, any solution, even an absolutely correct one, of any equation turns into a lottery - 50/50.

How could we get caught while solving a seemingly elementary example? And here it is at the moment of potentiation. The logarithms are gone, and with them all the limitations.

What to do in such a case? Refuse to eliminate logarithms? And completely abandon the solution of this equation?

No, we just, like real heroes from one famous song, will go around!

Before proceeding with the solution of any logarithmic equation, we will write down the ODZ. But after that, you can do whatever your heart desires with our equation. Having received the answer, we simply throw out those roots that are not included in our ODZ, and write down the final version.

Now let's decide how to write the ODZ. To do this, we carefully examine the original equation and look for suspicious places in it, such as division by x, the root of an even degree, etc. Until we have solved the equation, we do not know what x is equal to, but we know for sure that such x, which, when substituting, will give a division by 0 or the extraction of the square root of a negative number, are obviously not suitable for the answer. Therefore, such x's are unacceptable, while the rest will constitute the ODZ.

Let's use the same equation again:

log 3 (x 2 -3) = log 3 (2x)

As you can see, there is no division by 0, square roots also not, but there are expressions with x in the body of the logarithm. We immediately recall that the expression inside the logarithm must always be > 0. This condition is written in the form of ODZ:

Those. we have not solved anything yet, but we have already written down a mandatory condition for the entire sublogarithmic expression. The curly brace means that these conditions must be met at the same time.

The ODZ is written down, but it is also necessary to solve the resulting system of inequalities, which we will do. We get the answer x > v3. Now we know for sure which x will not suit us. And then we start solving the logarithmic equation itself, which we did above.

Having received the answers x 1 \u003d 3 and x 2 \u003d -1, it is easy to see that only x1 \u003d 3 is suitable for us, and we write it down as the final answer.

For the future, it is very important to remember the following: we solve any logarithmic equation in 2 stages. The first - we solve the equation itself, the second - we solve the condition of the ODZ. Both stages are performed independently of each other and are compared only when writing the answer, i.e. we discard all unnecessary and write down the correct answer.

To consolidate the material, we strongly recommend watching the video:

In the video, other examples of solving the log. equations and working out the method of intervals in practice.

To this on the subject, how to solve logarithmic equations until everything. If something according to the decision of the log. equations remained unclear or incomprehensible, write your questions in the comments.

Note: The Academy of Social Education (KSUE) is ready to accept new students.

Examples:

\(\log_(2)(⁡x) = 32\)
\(\log_3⁡x=\log_3⁡9\)
\(\log_3⁡((x^2-3))=\log_3⁡((2x))\)
\(\log_(x+1)((x^2+3x-7))=2\)
\(\lg^2⁡((x+1))+10=11 \lg⁡((x+1))\)

How to solve logarithmic equations:

When solving a logarithmic equation, you need to strive to convert it to the form \(\log_a⁡(f(x))=\log_a⁡(g(x))\), and then make the transition to \(f(x)=g(x) \).

\(\log_a⁡(f(x))=\log_a⁡(g(x))\) \(⇒\) \(f(x)=g(x)\).

Example:\(\log_2⁡(x-2)=3\)

Decision:
\(\log_2⁡(x-2)=\log_2⁡8\)
\(x-2=8\)
\(x=10\)
Examination:\(10>2\) - suitable for ODZ
Answer:\(x=10\)

ODZ:
\(x-2>0\)
\(x>2\)

Very important! This transition can only be made if:

You wrote for the original equation, and at the end check if the found ones are included in the DPV. If this is not done, extra roots may appear, which means the wrong decision.

The number (or expression) is the same on the left and right;

The logarithms on the left and right are "pure", that is, there should not be any, multiplications, divisions, etc. - only lone logarithms on both sides of the equals sign.

For example:

Note that equations 3 and 4 can be easily solved by applying the desired properties of logarithms.

Example . Solve the equation \(2\log_8⁡x=\log_8⁡2.5+\log_8⁡10\)

Decision :

		Let's write ODZ: \(x>0\).
\(2\log_8⁡x=\log_8⁡2,5+\log_8⁡10\) ODZ: \(x>0\)		On the left in front of the logarithm is the coefficient, on the right is the sum of the logarithms. This bothers us. Let's transfer the two to the exponent \(x\) by the property: \(n \log_b(⁡a)=\log_b⁡(a^n)\). We represent the sum of logarithms as a single logarithm by the property: \(\log_a⁡b+\log_a⁡c=\log_a(⁡bc)\)
\(\log_8⁡(x^2)=\log_8⁡25\)		We brought the equation to the form \(\log_a⁡(f(x))=\log_a⁡(g(x))\) and wrote down the ODZ, which means that we can make the transition to the form \(f(x)=g(x)\ ).
		Happened . We solve it and get the roots.
\(x_1=5\) \(x_2=-5\)		We check whether the roots fit under the ODZ. To do this, in \(x>0\) instead of \(x\) we substitute \(5\) and \(-5\). This operation can be performed orally.
\(5>0\), \(-5>0\)		The first inequality is true, the second is not. So \(5\) is the root of the equation, but \(-5\) is not. We write down the answer.

Answer : \(5\)

Example : Solve the equation \(\log^2_2⁡(x)-3 \log_2(⁡x)+2=0\)

Decision :

		Let's write ODZ: \(x>0\).
\(\log^2_2⁡(x)-3 \log_2(⁡x)+2=0\) ODZ: \(x>0\)		A typical equation solved with . Replace \(\log_2⁡x\) with \(t\).
\(t=\log_2⁡x\)
		Received the usual. Looking for its roots.
\(t_1=2\) \(t_2=1\)		Making a reverse substitution
\(\log_2(⁡x)=2\) \(\log_2(⁡x)=1\)		We transform the right parts, representing them as logarithms: \(2=2 \cdot 1=2 \log_2⁡2=\log_2⁡4\) and \(1=\log_2⁡2\)
\(\log_2(⁡x)=\log_2⁡4\) \(\log_2(⁡x)=\log_2⁡2 \)		Now our equations are \(\log_a⁡(f(x))=\log_a⁡(g(x))\) and we can jump to \(f(x)=g(x)\).
\(x_1=4\) \(x_2=2\)		We check the correspondence of the roots of the ODZ. To do this, instead of \(x\) we substitute \(4\) and \(2\) into the inequality \(x>0\).
\(4>0\) \(2>0\)		Both inequalities are true. So both \(4\) and \(2\) are the roots of the equation.

Answer : \(4\); \(2\).

basic properties.

logax + logay = log(x y);
logax − logay = log(x: y).

same grounds

log6 4 + log6 9.

Now let's complicate the task a little.

Examples of solving logarithms

What if there is a degree in the base or argument of the logarithm? Then the exponent of this degree can be taken out of the sign of the logarithm according to the following rules:

Of course, all these rules make sense if the ODZ logarithm is observed: a > 0, a ≠ 1, x >

Task. Find the value of the expression:

Transition to a new foundation

Let the logarithm logax be given. Then for any number c such that c > 0 and c ≠ 1, the equality is true:

Task. Find the value of the expression:

Basic properties of the logarithm

1.
2.
3.
4.
5.
6.
7.
8.
9.
10.
11.
12.
13.
14.
15.

The exponent is 2.718281828…. To remember the exponent, you can study the rule: the exponent is 2.7 and twice the year of birth of Leo Tolstoy.

Basic properties of logarithms

Knowing this rule, you will know both the exact value of the exponent and the date of birth of Leo Tolstoy.

Examples for logarithms

Take the logarithm of expressions

Example 1
a). x=10ac^2 (a>0, c>0).

By properties 3,5 we calculate

4. where .

Example 2 Find x if

Example 3. Let the value of logarithms be given

Calculate log(x) if

Basic properties of logarithms

Logarithms, like any number, can be added, subtracted and converted in every possible way. But since logarithms are not quite ordinary numbers, there are rules here, which are called basic properties.

These rules must be known - no serious logarithmic problem can be solved without them. In addition, there are very few of them - everything can be learned in one day. So let's get started.

Addition and subtraction of logarithms

Consider two logarithms with the same base: logax and logay. Then they can be added and subtracted, and:

logax + logay = log(x y);
logax − logay = log(x: y).

So, the sum of the logarithms is equal to the logarithm of the product, and the difference is the logarithm of the quotient. Please note: the key point here is - same grounds. If the bases are different, these rules do not work!

These formulas will help calculate the logarithmic expression even when its individual parts are not considered (see the lesson "What is a logarithm"). Take a look at the examples and see:

Since the bases of logarithms are the same, we use the sum formula:
log6 4 + log6 9 = log6 (4 9) = log6 36 = 2.

Task. Find the value of the expression: log2 48 − log2 3.

The bases are the same, we use the difference formula:
log2 48 − log2 3 = log2 (48: 3) = log2 16 = 4.

Task. Find the value of the expression: log3 135 − log3 5.

Again, the bases are the same, so we have:
log3 135 − log3 5 = log3 (135: 5) = log3 27 = 3.

As you can see, the original expressions are made up of "bad" logarithms, which are not considered separately. But after transformations quite normal numbers turn out. Many tests are based on this fact. Yes, control - similar expressions in all seriousness (sometimes - with virtually no changes) are offered at the exam.

Removing the exponent from the logarithm

It is easy to see that the last rule follows their first two. But it's better to remember it anyway - in some cases it will significantly reduce the amount of calculations.

Of course, all these rules make sense if the ODZ logarithm is observed: a > 0, a ≠ 1, x > 0. And one more thing: learn to apply all formulas not only from left to right, but also vice versa, i.e. you can enter the numbers before the sign of the logarithm into the logarithm itself. This is what is most often required.

Task. Find the value of the expression: log7 496.

Let's get rid of the degree in the argument according to the first formula:
log7 496 = 6 log7 49 = 6 2 = 12

Task. Find the value of the expression:

Note that the denominator is a logarithm whose base and argument are exact powers: 16 = 24; 49 = 72. We have:

I think the last example needs clarification. Where have logarithms gone? Until the very last moment, we work only with the denominator.

Formulas of logarithms. Logarithms are examples of solutions.

They presented the base and the argument of the logarithm standing there in the form of degrees and took out the indicators - they got a “three-story” fraction.

Now let's look at the main fraction. The numerator and denominator have the same number: log2 7. Since log2 7 ≠ 0, we can reduce the fraction - 2/4 will remain in the denominator. According to the rules of arithmetic, the four can be transferred to the numerator, which was done. The result is the answer: 2.

Transition to a new foundation

Speaking about the rules for adding and subtracting logarithms, I specifically emphasized that they only work with the same bases. What if the bases are different? What if they are not exact powers of the same number?

Formulas for transition to a new base come to the rescue. We formulate them in the form of a theorem:

Let the logarithm logax be given. Then for any number c such that c > 0 and c ≠ 1, the equality is true:

In particular, if we put c = x, we get:

It follows from the second formula that it is possible to interchange the base and the argument of the logarithm, but in this case the whole expression is “turned over”, i.e. the logarithm is in the denominator.

These formulas are rarely found in ordinary numerical expressions. It is possible to evaluate how convenient they are only when solving logarithmic equations and inequalities.

However, there are tasks that cannot be solved at all except by moving to a new foundation. Let's consider a couple of these:

Task. Find the value of the expression: log5 16 log2 25.

Note that the arguments of both logarithms are exact exponents. Let's take out the indicators: log5 16 = log5 24 = 4log5 2; log2 25 = log2 52 = 2log2 5;

Now let's flip the second logarithm:

Since the product does not change from permutation of factors, we calmly multiplied four and two, and then figured out the logarithms.

Task. Find the value of the expression: log9 100 lg 3.

The base and argument of the first logarithm are exact powers. Let's write it down and get rid of the indicators:

Now let's get rid of the decimal logarithm by moving to a new base:

Basic logarithmic identity

Often in the process of solving it is required to represent a number as a logarithm to a given base. In this case, the formulas will help us:

In the first case, the number n becomes the exponent in the argument. The number n can be absolutely anything, because it's just the value of the logarithm.

The second formula is actually a paraphrased definition. It's called like this:

Indeed, what will happen if the number b is raised to such a degree that the number b in this degree gives the number a? That's right: this is the same number a. Read this paragraph carefully again - many people “hang” on it.

Like the new base conversion formulas, the basic logarithmic identity is sometimes the only possible solution.

Task. Find the value of the expression:

Note that log25 64 = log5 8 - just took out the square from the base and the argument of the logarithm. Given the rules for multiplying powers with the same base, we get:

If someone is not in the know, this was a real task from the Unified State Examination 🙂

Logarithmic unit and logarithmic zero

In conclusion, I will give two identities that are difficult to call properties - rather, these are consequences from the definition of the logarithm. They are constantly found in problems and, surprisingly, create problems even for "advanced" students.

logaa = 1 is. Remember once and for all: the logarithm to any base a from that base itself is equal to one.
loga 1 = 0 is. The base a can be anything, but if the argument is one, the logarithm is zero! Because a0 = 1 is a direct consequence of the definition.

That's all the properties. Be sure to practice putting them into practice! Download the cheat sheet at the beginning of the lesson, print it out and solve the problems.

Basic properties of the logarithm

The above properties need to be known, since, on their basis, almost all problems and examples are solved based on logarithms. The remaining exotic properties can be derived by mathematical manipulations with these formulas

1.
2.
3.
4.
5.
6.
7.
8.
9.
10.
11.
12.
13.
14.
15.

When calculating the formulas for the sum and difference of logarithms (3.4) are encountered quite often. The rest are somewhat complex, but in a number of tasks they are indispensable for simplifying complex expressions and calculating their values.

Common cases of logarithms

Some of the common logarithms are those in which the base is even ten, exponential or deuce.
The base ten logarithm is usually called the base ten logarithm and is simply denoted lg(x).

It can be seen from the record that the basics are not written in the record. For example

The natural logarithm is the logarithm whose basis is the exponent (denoted ln(x)).

The exponent is 2.718281828…. To remember the exponent, you can study the rule: the exponent is 2.7 and twice the year of birth of Leo Tolstoy. Knowing this rule, you will know both the exact value of the exponent and the date of birth of Leo Tolstoy.

And another important base two logarithm is

The derivative of the logarithm of the function is equal to one divided by the variable

The integral or antiderivative logarithm is determined by the dependence

The above material is enough for you to solve a wide class of problems related to logarithms and logarithms. For the sake of understanding the material, I will give only a few common examples from school curriculum and universities.

Examples for logarithms

Take the logarithm of expressions

Example 1
a). x=10ac^2 (a>0, c>0).

By properties 3,5 we calculate

2.
By the difference property of logarithms, we have

3.
Using properties 3.5 we find

4. where .

A seemingly complex expression using a series of rules is simplified to the form

Finding Logarithm Values

Example 2 Find x if

Decision. For the calculation, we apply properties 5 and 13 up to the last term

Substitute in the record and mourn

Since the bases are equal, we equate the expressions

Logarithms. First level.

Let the value of the logarithms be given

Calculate log(x) if

Solution: Take the logarithm of the variable to write the logarithm through the sum of the terms

This is just the beginning of acquaintance with logarithms and their properties. Practice calculations, enrich your practical skills - you will soon need the acquired knowledge to solve logarithmic equations. Having studied the basic methods for solving such equations, we will expand your knowledge for another equally important topic - logarithmic inequalities ...

Basic properties of logarithms

These rules must be known - no serious logarithmic problem can be solved without them. In addition, there are very few of them - everything can be learned in one day. So let's get started.

Addition and subtraction of logarithms

Consider two logarithms with the same base: logax and logay. Then they can be added and subtracted, and:

logax + logay = log(x y);
logax − logay = log(x: y).

These formulas will help calculate the logarithmic expression even when its individual parts are not considered (see the lesson "What is a logarithm"). Take a look at the examples and see:

Task. Find the value of the expression: log6 4 + log6 9.

Since the bases of logarithms are the same, we use the sum formula:
log6 4 + log6 9 = log6 (4 9) = log6 36 = 2.

Task. Find the value of the expression: log2 48 − log2 3.

The bases are the same, we use the difference formula:
log2 48 − log2 3 = log2 (48: 3) = log2 16 = 4.

Task. Find the value of the expression: log3 135 − log3 5.

Again, the bases are the same, so we have:
log3 135 − log3 5 = log3 (135: 5) = log3 27 = 3.

Removing the exponent from the logarithm

Now let's complicate the task a little. What if there is a degree in the base or argument of the logarithm? Then the exponent of this degree can be taken out of the sign of the logarithm according to the following rules:

It is easy to see that the last rule follows their first two. But it's better to remember it anyway - in some cases it will significantly reduce the amount of calculations.

How to solve logarithms

This is what is most often required.

Task. Find the value of the expression: log7 496.

Let's get rid of the degree in the argument according to the first formula:
log7 496 = 6 log7 49 = 6 2 = 12

Task. Find the value of the expression:

Note that the denominator is a logarithm whose base and argument are exact powers: 16 = 24; 49 = 72. We have:

I think the last example needs clarification. Where have logarithms gone? Until the very last moment, we work only with the denominator. They presented the base and the argument of the logarithm standing there in the form of degrees and took out the indicators - they got a “three-story” fraction.

Transition to a new foundation

Formulas for transition to a new base come to the rescue. We formulate them in the form of a theorem:

Let the logarithm logax be given. Then for any number c such that c > 0 and c ≠ 1, the equality is true:

In particular, if we put c = x, we get:

These formulas are rarely found in ordinary numerical expressions. It is possible to evaluate how convenient they are only when solving logarithmic equations and inequalities.

However, there are tasks that cannot be solved at all except by moving to a new foundation. Let's consider a couple of these:

Task. Find the value of the expression: log5 16 log2 25.

Note that the arguments of both logarithms are exact exponents. Let's take out the indicators: log5 16 = log5 24 = 4log5 2; log2 25 = log2 52 = 2log2 5;

Now let's flip the second logarithm:

Since the product does not change from permutation of factors, we calmly multiplied four and two, and then figured out the logarithms.

Task. Find the value of the expression: log9 100 lg 3.

The base and argument of the first logarithm are exact powers. Let's write it down and get rid of the indicators:

Now let's get rid of the decimal logarithm by moving to a new base:

Basic logarithmic identity

Often in the process of solving it is required to represent a number as a logarithm to a given base. In this case, the formulas will help us:

In the first case, the number n becomes the exponent in the argument. The number n can be absolutely anything, because it's just the value of the logarithm.

The second formula is actually a paraphrased definition. It's called like this:

Like the new base conversion formulas, the basic logarithmic identity is sometimes the only possible solution.

Task. Find the value of the expression:

Note that log25 64 = log5 8 - just took out the square from the base and the argument of the logarithm. Given the rules for multiplying powers with the same base, we get:

If someone is not in the know, this was a real task from the Unified State Examination 🙂

Logarithmic unit and logarithmic zero

logaa = 1 is. Remember once and for all: the logarithm to any base a from that base itself is equal to one.
loga 1 = 0 is. The base a can be anything, but if the argument is one, the logarithm is zero! Because a0 = 1 is a direct consequence of the definition.

That's all the properties. Be sure to practice putting them into practice! Download the cheat sheet at the beginning of the lesson, print it out and solve the problems.